高等数学英文版课件PPT 08 Further Applications of Integration.ppt 127页

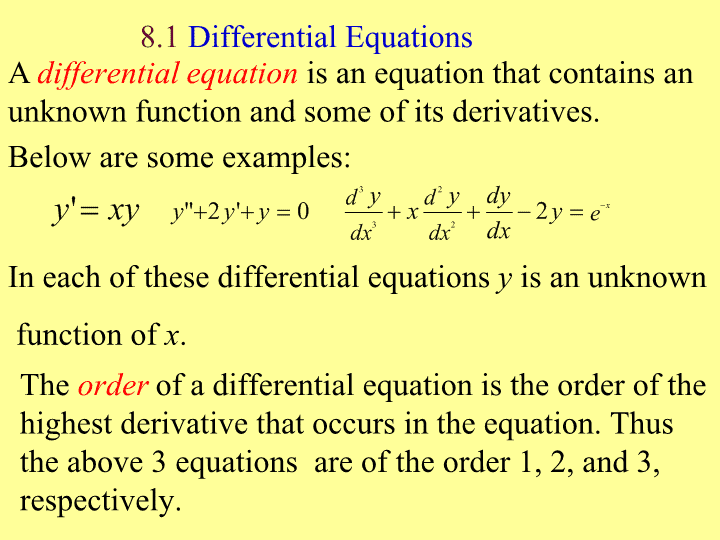
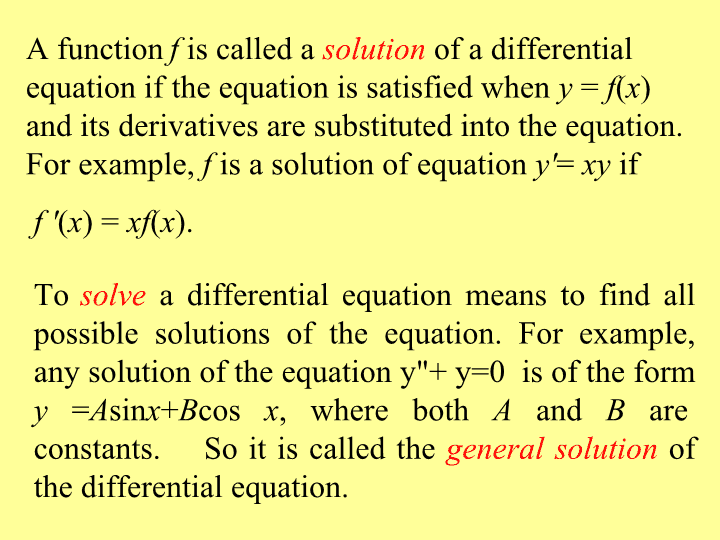
'Chapter8FurtherApplicationsofIntegration Adifferentialequationisanequationthatcontainsanunknownfunctionandsomeofitsderivatives.Belowaresomeexamples:Theorderofadifferentialequationistheorderofthehighestderivativethatoccursintheequation.Thustheabove3equationsareoftheorder1,2,and3,respectively.Ineachofthesedifferentialequationsyisanunknownfunctionofx.8.1DifferentialEquations Tosolveadifferentialequationmeanstofindallpossiblesolutionsoftheequation.Forexample,anysolutionoftheequationy"+y=0isoftheformy=Asinx+Bcosx,wherebothAandBareconstants.Soitiscalledthegeneralsolutionofthedifferentialequation.Afunctionfiscalledasolutionofadifferentialequationiftheequationissatisfiedwheny=f(x)anditsderivativesaresubstitutedintotheequation.Forexample,fisasolutionofequationy"=xyiff"(x)=xf(x). AnyparticularsolutionsareobtainedbysubstitutingvaluesforthearbitraryconstantsAandB.Forinstance,y=sinxisaparticularsolutionoftheabovedifferentialequationbychoosingA=1,B=0inthegeneralsolution.Ingeneral,solvingadifferentialequationisnotaneasymatter. SeparableequationAseparableequationisafirst-orderdifferentialequationthatcanbewrittenintheformdy/dx=g(x)f(y).Thenameseparablecomesfromthefactthattheexpressionontherightsidecanbe“separated”intoafunctionofxandafunctionofy.Equivalently,wecouldwrite sothatally’sareononesideoftheequationandallx’sareontheotherside.Thenweintegratebothsidesoftheequation:Tosolvethisequationwerewriteitinthedifferentialformh(y)dy=g(x)dxItdefinesyimplicitlyasafunctionofx.Insomecaseswemaybeabletosolveforyintermsofx ThejustificationoftheabovelaststepcomesformtheSubstitutionRule: SolvethedifferentialequationSolutionWritingtheequationindifferentialformandintegratingbothsides,wehaveExample1 whereCisanarbitraryconstant.(WecouldhaveusedaconstantC1ontheleftsideandanotherconstantC2ontherightside,butthenwecouldcombinetheseconstantsbywritingC=C2-C1Theabovegeneralsolutionisinimplicitform.Inthiscaseitisimpossibletoexpressyexplicitlyasafunctionofx. SolvethedifferentialequationSolutionRewritetheequationusingLeibniznotation:Ify0,wecanrewriteitindifferentialnotationandintegrate:dy/dx=x2y.Example2dy/y=x2dx,y0,ln|y|=x3/3+C Notethatthefunctiony=0isalsoasolutionofthegivendifferentialequation.SothegeneralsolutionisintheformInthiscase,wecansolveexplicitlyfory:whereAisanarbitraryconstant(A=eCor0). Initial-valueproblemTheproblemoffindingasolutionofthedifferentialequationthatsatisfiestheinitialconditioniscalledaninitial-valueproblem.Inmanyphysicalproblemsweneedtofindtheparticularsolutionthatsatisfiesaconditionoftheformy(x0)=y0.Thisiscalledaninitialcondition. Example1SolvethedifferentialequationSolutionWritethedifferentialequationas:Integratebothsides:xdy/dx=-yordy/y=-dx/x.ln|y|=-ln|x|+C,|y|=1/|x|eC TodetermineKweputx=4andy=2inthisequation:2=K/4K=8Sothesolutionoftheinitial-valueproblemisy=8/x,x>0 Example2Findthesolutionofdy/dx=6x2/(2y+cosy)thatsatisfiesy(1)=.SolutionFromExample1inthelastpart,weknowthatthegeneralsolutionisy2+siny=2x3+C Therefore,thesolutionisgivenimplicitlybyy2+siny=2x3+2–2 Example3Solvey"=1+y2-2x-2xy2,y(0)=0,andgraphthesolution.Substitutingx=0andy=0inthisequation,wegetC=0.SoSolutionFactortherightsideastheproductofafunctionofxandafunctionofy:tan-1y=x-x2 Tographthisequation,noticethatitisequivalenttoy=tan(x-x2)providedthat-/20,thisshowsthatd2y/dx2<0exceptwhencos=1.Thusthecycloidisconcavedownwardontheintervals(2n,2(n+1)).Asimilarcomputationshowsthatdy/dx-as2n-–,soindeedthereareverticaltangentswhen=2n,thatis,whenx=2nr. Example2AcurveCisdefinedbyx=t2andy=t3-3t.(a)ShowthatChastwotangentsat(3,0)andfindtheirequations.(b)FindthepointsonCwherethetangentishorizontalorvertical.(c)Determinewherethecurverisesorfallsandwhereitisconcaveupwardordownward.(d)Sketchthecurve. Ifthecurveisgivenbyparametricequationsx=f(t)andy=g(t),t,thenwecanadapttheearlierformulabyusingtheSubstitutionRuleforDefiniteIntegralsasfollows:(2)AreasTheareaunderacurvey=F(x)fromatobiswhereF(x)0. Example1Findtheareaunderonearchofthecycloidx=r(-sin),y=r(1-cos).UsingtheSubstitutionRulewithy=r(1-cos)anddx=r(1-cos)d,wehaveSolutionOnearchofthecycloidisgivenby Example2Findtheareaoftheregionenclosedbytheloopofthecurvedefinedbyx=t2andy=t3-3t.(thesameasthatinExample2ofthefirstpartofthissection). Thepointontheloopwherethecurvecrossesitselfis(3,0),thecorrespondingparametervaluesare.Theareaoftheloopisobtainedbysubtractingtheareaunderthebottompartoftheloopfromtheareaunderthetoppartoftheloop.Solution 8.7PolarCoordinatesAcoordinatesystemrepresentsapointintheplanebyanorderedpairofnumberscalledcoordinates.SofarwehavebeenusingCartesiancoordinates,whicharedirecteddistancesfromtwoperpendicularaxes.Nowwedescribeacoordinatesystemcalledthepolarcoordinatesystem,whichismoreconvenientformanypurposes.Wechooseapointintheplanethatiscalledthepole(ororigin)andlabeledO.Thenwedrawaray(half-line)startingatOcalledthepolaraxis.Thisaxisisusuallydrawnhorizontallytotherightandcorrespondstothepositivex-axisinCartesiancoordinates. IfPisanyotherpointintheplane,letrbethedistancefromOtoPandletbetheangle(usuallymeasuredinradians)betweenthepolaraxisandthelineOPasinthefigure.ThenthepointPisrepresentedbytheorderedpair(r,)andr,arecalledpolarcoordinatesofP. Weusetheconventionthatanangleispositiveifmeasuredinthecounterclockwisedirectionfromthepolaraxisandnegativeintheclockwisedirection.IfP=O,thenr=0andweagreethat(0,)representsthepoleforanyvalueof. Weextendthemeaningofpolarcoordinates(r,)tothecaseinwhichrisnegativebyagreeingthatthepoints(-r,)and(r,)liesonthesamelinethroughOandatthesamedistance|r|fromO,butontheoppositesidesofO.Ifr>0,thepoint(r,)liesinthesamequadrantas;ifr<0,thepoint(r,)liesinthequadrantontheoppositesideofthepole. Noticethat(-r,)representsthesamepointas(r,+).Infact,sinceacompletecounterclockwiserotationisgivenbyanangle2,thepointrepresentedbypolarcoordinates(r,)isalsorepresentedby(r,+2n)and(-r,+(2n+1)) Example1Plotthepointswhosepolarcoordinatesaregiven(a)(1,5/4)(b)(2,3)(c)(2,-2/3)(d)(-3,3/4)SolutionThepointsareplottedinthefigure.Inpart(d)thepoint(-3,3/4)islocatedthreeunitsfromthepoleinthefourthquadrantbecausetheangle3/4isinthesecondquadrantandr=-3isnegative. TheconnectionbetweenpolarandCartesiancoordinatescanbeseenfromthefigure,inwhichthepolecorrespondstotheoriginandthepolaraxiscoincideswiththepositivex-axis.IfthepointPhasCartesiancoordinates(x,y)andpolarcoordinates(r,),thencos=x/r,sin=y/randsox=rcos,y=rsinAlthoughtheaboveequationswerededucedfromthefigure,whichillustratesthecasewherer>0and0<</2,theseequationsarevalidforallvaluesofrand. WecanusetheaboveformulatofindtheCartesiancoordinatesofapointwhenthepolarcoordinatesareknown.WecanalsousethebelowequationstofindrandiftheCartesiancoordinatesofapointareknown:r2=x2+y2tan=y/xNoticethattheaboveequationsdonotuniquelydeterminewhenxandyaregiven,becauseasincreasesthroughtheinterval0<2,eachvalueoftanoccurstwice.SeethenextpageTherefore,inconvertingfromCartesiantopolarcoordinates,itisnotgoodenoughjusttofindrandthatsatisfytheequations,wemustchoosesothatthepoint(r,)liesinthecorrectquadrant. Example2Convertthepoint(2,/3)frompolartoCartesiancoordinates.SolutionSincer=2and=/3, Sincethepoint(1,-1)liesinthefourthquadrant,wecanchoose=-/4or=7/4.Thusonepossibleansweris(2,-/4).Anotheris(2,7/4).Example3RepresentthepointwithCartesiancoordinates(1,-1)intermsofpolarcoordinates.SolutionIfwechoosertobepositive,then TheGraphofaPolarEquationThegraphofapolarequationr=f(),ormoregenerallyF(r,)=0,consistsofallpointsPthathaveatleastonepolarrepresentation(r,)whosecoordinatessatisfytheequation. Example1Whatcurveisrepresentedbythepolarequationr=2.SolutionThecurveconsistsofallpoints(r,)withr=2.Sincerrepresentsthedistancefromthepointtothepole,thecurver=2representsthecirclewithcenterOandradius2.Ingeneral,theequationr=arepresentsacirclewithcenterOradius|a|. SolutionThiscurveconsistsofallpoints(r,)suchthatthepolarangleis1radian.ItisthestraightlinethatpassesthroughOandmakesanangleof1radianwiththepolaraxis.Example2Sketchthepolarcurve=1.Noticethatthepoints(r,1)onthelinewithr>0areinthefirstquadrant,whereasthosewithr<0areinthethirdquadrant.(,+1)2 Example3(a)Sketchthecurvewithpolarequationr=2cos.(b)FindaCartesianequationforthiscurve.Solution(a)Wefindthevaluesofrforsomeconvenientvaluesofandplotthecorrespondingpoints(r,).Thenwejointhesepointstosketchthecurve,whichappearstobeacircle.Wehaveusedonlyvaluesofbetween0and,sinceifweletincreasesbeyond,weobtainthesamepointsagain. (b)Multiplyrtobothsidesoftheequationr=2cos:r2=2rcos,x2+y2=2x,x2+y2-2x=0Completingthesquare,weobtain(x-1)2+y2=1whichistheequationofacirclewithcenter(1,0)andradius1.Thefigurebelowshowsageometricalillustrationthatthecirclehastheequationr=2cos.TheangleOPQisarightangleandsor/2=cos. Example4Sketchthecurver=1+sin.SolutionFirstsketchthegraphofr=1+sininCartesiancoordinatesbyshiftingthesinecurveuponeunit.Thisenablesustoreadataglancethevaluesofrthatcorrespondtoincreasingvaluesof.Weseethatasincreasesfrom0to/2,rincreasefrom1to2;asincreasesfrom/2to,rdecreasefrom2to1;asincreasesfromto3/2,rdecreasefrom1to0;asincreasesfrom3/2to2,rincreasefrom0to1.Ifweletincreasesbeyond2ordecreasebeyond0,wewouldsimplyretraceourpath.Thenwesketchoutthecompletecurveasinthefigure.Itiscalledacardioidbecauseitisshapedlikeaheart.. Example5Sketchthecurvewithpolarequationr=cos2.SolutionWefirstsketchr=cos2,0<2,inCartesiancoordinates.Asincreasesfrom0to/4,rdecreasefrom1to0,andsowedrawthecorrespondingportionofthepolarcurve.Asincreasesfrom/4to/2,rdecreasefrom0to–1.ThismeansthatthedistancefromOincreasesfrom0to1,butinsteadofbeinginthefirstquadrant,thisportionofthepolarcurveliesontheoppositesideofthepoleinthethirdquadrant.Theremainderofthecurveisdrawninasimilarfashion.Theresultingcurvehasfourloopsandiscalledafour-leavedrose. Whensketchingpolarcurvesitissometimeshelpfultotakeadvantageofsymmetry.Thefollowingarethreerules.Ifapolarequationisunchangedwhenisreplacedby-.Thecurveissymmetricaboutthepolaraxis.Ifapolarequationisunchangedwhenrisreplacedby-r.Thecurveissymmetricaboutthepole.Ifapolarequationisunchangedwhenisreplacedby-.Thecurveissymmetricabouttheverticalline=/2. ThecurvesketchedinExamples3and5aresymmetricaboutthepolaraxis.ThecurvesinExample4and5aresymmetricabout=π/2.Thefour-leavedroseisalsosymmetricaboutthepole.Thesesymmetrypropertiescouldbeusedinsketchingcurves.Weonlyneedtoplotapartofthecurveandthenapplythesymmetry. TangentstopolarcurvesTofindatangentlinetoapolarcurver=f()weregardasaparameterandwriteitsparametricequationsx=f()cos,y=f()sin.ThenusingthemethodforfindingslopesofparametriccurveswehaveWelocatehorizontaltangentsbyfindingthepointswhendy/d=0(providedthatdx/d0).Welocateverticaltangentsatthepointswhendx/d=0(providedthatdy/d0). Noticethatifwearelookingfortangentlinesatthepole,thenr=0andtheaboveequationsimplifiestoifForinstance,inExample5,wefoundthatr=cos2=0when=/4or3/4.Thismeansthatthelines=/4and=3/4or(y=xandy=-xaretangentlinestor=cos2attheorigin. ExampleForthecardioidr=1+sin,findtheslopeofthetangentlinewhen=/3.Findthepointsonthecardioidwherethetangentlineishorizontalorvertical.orSolutionUsingthederivedformulawithr=1+sin,wehave Insteadofmemorizingtheformula,wecouldemploythemethodusedtoderivetheformula: (a)Theslopeofthetangentatthepointwhere=/3is (b)ObservethatTherefore,therearehorizontaltangentsatthepoints(2,/2),(1/2,7/6),(1/2,11/6)andverticaltangentsat(3/2,/6).(3/2,5/6).When=3/2,bothandare0,sowemustbecareful.Usingl’Hospital’sRule,wehaveBysymmetry,Thusthereisaverticaltangentlineatthepole.r=1+sin Page55817Solution ++–+––––xy–++–curve'