高等数学英文版课件PPT 01 Limits and Rates of Change.ppt 41页

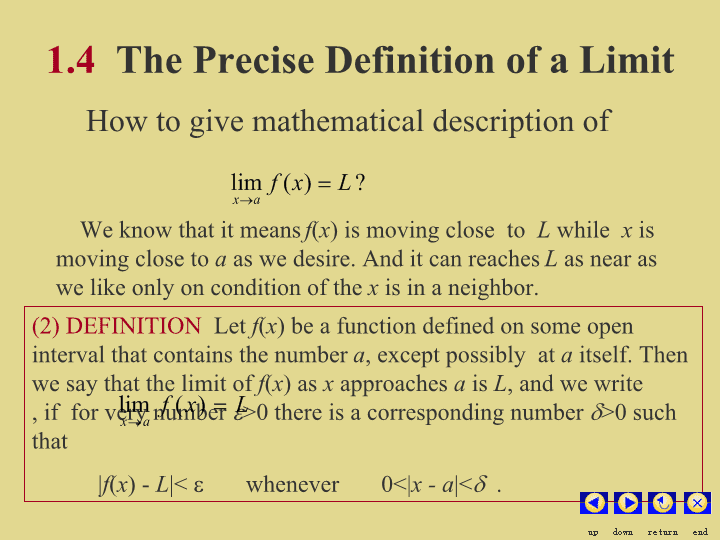
'Chapter0neLimitsandRatesofChangeupdownreturnend 1.4ThePreciseDefinitionofaLimitWeknowthatitmeansf(x)ismovingclosetoLwhilexismovingclosetoaaswedesire.AnditcanreachesLasnearaswelikeonlyonconditionofthexisinaneighbor.(2)DEFINITIONLetf(x)beafunctiondefinedonsomeopenintervalthatcontainsthenumbera,exceptpossiblyataitself.Thenwesaythatthelimitoff(x)asxapproachesaisL,andwewrite,ifforverynumber>0thereisacorrespondingnumber>0suchthat|f(x)-L|<whenever0<|x-a|<.updownreturnendHowtogivemathematicaldescriptionof Inthedefinition,themainpartisthatforarbitrarily>0,thereexistsa>0suchthatifallxthat0<|x-a|<then|f(x)-L|<.Anothernotationforisf(x)Lasxa.Geometricinterpretationoflimitscanbegivenintermsofthegraphofthefunctiony=L+y=L-y=Laa-a+oxy=f(x)yupdownreturnend Example1ProvethatSolutionLetbeagivenpositivenumber,wewanttofindapositivenumbersuchthat|(4x-5)-7|<whenever0<|x-3|<.But|(4x-5)-7|=4|x-3|.Therefore4|x-3|<whenever0<|x-3|<.Thatis,|x-3|</4whenever0<|x-3|<.Example2ProvethatExample3Provethatupdownreturnend Example4ProvethatSimilarlywecangivethedefinitionsofone-sidedlimitsprecisely.(4)DEFINITIONOFLEFT-SIDEDLIMITIfforeverynumber>0thereisacorrespondingnumber>0suchthat|f(x)-L|<whenever00thereisacorrespondingnumber>0suchthat|f(x)-L|<whenever00thereisacorrespondingnumber>0suchthatf(x)>Mwhenever0<|x-a|<.updownreturnend ExampleProvethatExample5Provethat(6)DEFINITIONLetf(x)beafunctiondefinedonsomeopenintervalthatcontainsthenumbera,exceptpossiblyataitself.Thenwesaythatthelimitoff(x)asxapproachesaisinfinity,andwewrite,ifforverynumberN<0thereisacorrespondingnumber>0suchthatf(x)0thereisacorrespondingnumber>0suchthat|f(x)-f(a)|<whenever|x-a|<.Notethat:(1)f(a)isdefined(2)exists.updownreturnend Exampleisdiscontinuousatx=2,sincef(2)isnotdefined.Exampleiscontinuousatx=2..ExampleProvethatsinxiscontinuousatx=a.(2)DefinitionAfunctionf(x)iscontinuousfromtherightateverynumberaifAfunctionf(x)iscontinuousfromtheleftateverynumberaifupdownreturnend (2)DefinitionAfunctionf(x)iscontinuousonanintervalifitiscontinuousateverynumberintheinterval.(atanendpointoftheintervalweunderstandcontinuoustomeancontinuousfromtherightorcontinuousfromtheleft)ExampleAteachintegern,thefunctionf(x)=[x]iscontinuousfromtherightanddiscontinuousfromtheleft.ExampleShowthatthefunctionf(x)=1-(1-x2)1/2iscontinuousontheinterval[-1,1].(4)TheoremIffunctionsf(x),g(x)iscontinuousataandcisaconstant,thenthefollowingfunctionsarecontinuousata:1.f(x)+g(x)2.f(x)-g(x)3.f(x)g(x)4.f(x)[g(x)]-1(g(a)isn’t0.)updownreturnend (5)THEOREM(a)anypolynomialiscontinuouseverywhere,thatis,itiscontinuousonR1=().(b)anyrationalfunctioniscontinuouswhereveritisdefined,thatis,itiscontinuousonitsdomain.ExampleFind(6)THEOREMIfnisapositiveeveninteger,thenf(x)=iscontinuouson[0,).Ifnisapositiveoddinteger,thenf(x)=iscontinuouson().ExampleOnwhatintervalsiseachfunctioncontinuous?updownreturnend (8)THEOREMIfg(x)iscontinuousataandf(x)iscontinuousatg(a)then(fog)(x))=f(g(x))iscontinuousata.(7)THEINTERMEDIATEVALUETHEOREMSupposethatf(x)iscontinuousontheclosedinterval[a,b].LetNbeanynumberstrictlybetweenf(a)andf(b).Thenthereexistsanumbercin(a,b)suchthatf(c)=Nyxby=Na(7)THEOREMIff(x)iscontinuousatband,thenupdownreturnend ExampleShowthatthereisarootoftheequation4x3-6x2+3x-2=0between1and2.updownreturnend 1.6Tangent,andOtherRatesofChangeA.Tangent(1)DefinitionTheTangentlinetothecurvey=f(x)atpointP(a,f(a))isthelinethroughPwithslopeprovidedthatthislimitexists.ExampleFindtheequationofthetangentlinetotheparabolay=x2atthepointP(1,1).updownreturnend B.OtherratesofchangeThedifferencequotientiscalledtheaverageratechangeofywithrespectxovertheinterval[x1,x2].(4)instantaneousrateofchange=atpointP(x1,f(x1))withrespecttox.Supposeyisaquantitythatdependsonanotherquantityx.Thusyisafunctionofxandwewritey=f(x).Ifxchangesfromx1andx2,thenthechangeinx(alsocalledtheincrementofx)isx=x2-x1andthecorrespondingchangeinyisx=f(x2)-f(x1).updownreturnend (1)whatisatangenttoacircle?Canwecopythedefinitionofthetangenttoacirclebyreplacingcirclebycurve?1.1ThetangentandvelocityproblemsThetangenttoacircleisalinewhichintersectsthecircleonceandonlyonce.Howtogivethedefinitionoftangentlinetoacurve?Forexample,updownreturnend Fig.(a)InFig.(b)therearestraightlineswhichtouchthegivencurve,buttheyseemtobedifferentfromthetangenttothecircle.L2Fig.(b)L1updownreturnend Letusseethetangenttoacircleasamovinglinetoacertainline:Sowecanthinkthetangenttoacurveisthelineapproachedbymovingsecantlines.PQupdownreturnendQ" xmPQ231.52.51.12.11.012.011.0012.001Example1:Findtheequationofthetangentlinetoaparabolay=x2atpoint(1,1).Qisapointonthecurve.Qy=x2Pupdownreturnend ThenwecansaythattheslopemofthetangentlineisthelimitoftheslopesmQPofthesecantslines.AndweexpressthissymbolicallybywritingAndSowecanguessthatslopeofthetangenttotheparabolaat(1,1)isveryclosedto2,actuallyitis2.Thentheequationofthetangentlinetotheparabolaisy-1=2(x-2)i.ey=2x-3.updownreturnend SupposethataballisdroppedfromtheupperobservationdeckoftheOrientalPearlTowerinShanghai,280mabovetheground.Findthevelocityoftheballafter5seconds.Fromphysicsweknowthatthedistancefallenaftertsecondsisdenotedbys(t)andmeasuredinmeters,sowehaves(t)=4.9t2.Howtofindthevelocityatt=5?(2)Thevelocityproblem:Solutionupdownreturnend Sowecanapproximatethedesiredquantitybycomputingtheaveragevelocityoverthebrieftimeintervalofthen-thofasecondfromt=5,suchas,thetenth,twenty-thandsoon.Thenwehavethetable:TimeintervalAveragevelocity(m/s)52orx<2),f(x)iscloseto4.Thenwecansaythat:thelimitofthefunctionf(x)=x2-x+2asxapproaches2isequalto4.Thenwegiveanotationforthis:Ingeneral,thefollowingnotation: (1)Definition:WewriteGuessthevalueof.Noticethatthefunctionisnotdefinedatx=1,andx<1f(x)x>1f(x)0.50.6666671.50.4000000.90.5263161.10.4761900.990.5025131.010.4975120.9990.5002501.0010.4997500.999.0.5000251.00010.499975Example1updownreturnendandsay“thelimitoff(x),asxapproachesa,equalsL”.SolutionIfwecanmakethevaluesoff(x)arbitrarilyclosetoL(asclosetoLaswelike)bytakingxtobesufficientlyclosetoabutnotequaltoa.Sometimesweusenotationf(x)Lasxa. Example1FindExample2FindNoticethatasxawhichmeansthatxapproachesa,xmay>aandxmay0.SowecannotsayH(x)approachesanumberasxa.updownreturnend One-sideLimits:EventhoughthereisnosinglenumberthatH(x)approachesastapproaches0.thatis,doesnotexist.Butastapproaches0fromleft,t<0,H(x)approaches0.Thenwecanindicatethissituationsymbolicallybywriting:Butastapproaches0fromright,t>0,H(x)approaches1.Thenwecanindicatethissituationsymbolicallybywriting:updownreturnend WewriteAndsaytheleft-handlimitoff(x)asxapproachesa(orthelimitoff(x)asxapproachesafromleft)isequaltoL.Thatis,wecanmakethevalueoff(x)arbitrarilyclosetoLbytakingxtobesufficientlyclosetoaandxlessthana.Andsaytheright-handlimitoff(x)asxapproachesa(orthelimitoff(x)asxapproachesafromright)isequaltoL.Thatis,wecanmakethevalueoff(x)arbitrarilyclosetoLbytakingxtobesufficientlyclosetoaandxgreaterthana.WewriteHerexa+”meansthatxapproachesaandx>a.(2)Definition:Herexa-”meansthatxapproachesaandx0)11.wherenisapositiveinteger,updownreturnend Example6.CalculateExample1.FindExample2.FindExample3.CalculateExample4.CalculateExample5.Calculatewhereupdownreturnend Iff(x)isapolynomialorrationalfunctionandaisinthedomainoff(x),then(1)THEOREMifandonlyifExample:ShowthatExample:If,determinewhetherexists.Example:Provethatdoesnotexists.Example:Provethatdoesnotexists,wherevalueof[x]isdefinedasthelargestintegerthatislessthanorequaltox.updownreturnend (2)THEOREMIff(x)g(x)forallxinanopenintervalthatcontainsa(exceptpossiblyata)andthelimitsoffandgexistasxapproachesa,then(3)SQUEEZETHEOREMIff(x)g(x)h(x)forallxinanopenintervalthatcontainsa(exceptpossiblyata)andthenExample:Showthatupdownreturnend'